Malgosia Ip, Mathematics & Statistics editor
“I really don’t think it’s possible,” I say again, unfolding the rumpled sheet of paper. I have been trying to solve one of Erik Demaine’s folding puzzles for a few hours now. Some of the creases have been folded so many times that the paper is starting to tear.
Demaine, a Canadian math prodigy and now Professor at MIT, has been inventing and solving puzzles with his father since he was six years old. When Demaine’s research took him down the path of computational origami, the puzzles followed suit, becoming paper-based folding puzzles. Now Erik and his dad’s folding puzzles have become a tradition at the MIT Computer Science and Artificial Intelligence Laboratory (CSAIL) annual meeting.
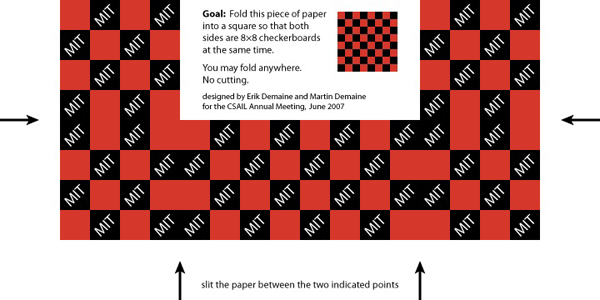
The neat thing about these puzzles is that each one illustrates an unsolved mathematical problem. The one I’m working on is from the 2007 meeting, and it’s one of Demaine’s favourites. (See image, above: The 2007 CSAIL puzzle created by Erik and Martin Demaine. Reproduced with permission.)
“It relates to a mathematical problem called ‘turning inside out’,” Demaine tells me. “What surfaces can be turned inside-out (reversing their inside and outside) by a continuous folding motion using [a finite number of] creases?” Knowing the problem that the puzzle represents is actually a clue to its solution.
Download the puzzle and join me on a trip down the mathematical origami rabbit-hole.
Fold the paper into a square…
The instructions are simple: fold the paper into a square so that both sides form perfect 8 x 8 checkerboards. No problem. I begin haphazardly by folding the paper into 4 x 4 grids and trying my luck. It almost seems like the first attempt works, but this puzzle plays tricks on the eyes. I soon realize that two black squares are touching in several places. I begin again, trying to do something different this time, but the result is very similar – some of the squares seem to work, but not all of it is correct. After several more attempts, I start to feel like I’m doing the same thing over and over. There must be a better way.

My first try: I had started by folding along all the 4 x 4 grids and thought I had it almost right away… but it was only half right.
Paper folding, or origami, has been around for fifteen centuries, and most of the iconic designs (paper crane, anyone?) arose from treating it like an art form, not a science. Origami masters developed a knowledge of the basic folding patterns and how to put them together to achieve certain shapes – it took much practice to develop a new pattern that looked nice.
It wasn’t until much later that mathematics was added to the origami toolkit.
Origami meets math
Beginning in the late-1980s, NASA physicist Robert Lang and Japanese biochemist Toshiyuki Meguro developed a systematic design method that could be used to build arbitrarily complicated origami structures. They called it the circle-river method because of the way the desired design is encoded – a circle for each flap of the final figure and a line or “river” for each connecting piece.
Combining this algorithm with the power of the computer, Lang was able to create new origami designs that were well beyond what a person with pencil and paper could do. This was the birth of computational origami, which brought people like Demaine onto the origami bandwagon.
“Computational origami is (in some sense) a subfield of computational geometry,” explains Demaine. He was looking for something to research for his PhD and had recently seen a paper by Lang published in a prominent computational geometry journal.
“[The field] was just getting started at that point (there were just two papers on the topic), and it sounded like a fun direction to explore. I’d never folded paper at that point.”
Since that seminal work, the power of mathematics has completely transformed the art of origami. In fact, Demaine recently published a paper describing a universal algorithm for folding any 3D structure that guarantees a minimum number of seams.
I’m sure a computer algorithm would be able to solve this puzzle with ease, but I am determined to get it myself. I decide to try and make some rules that can help me solve the folding puzzle. After all, there are a limited number of ways that this puzzle can be folded into an 8 x 8 square and, if I can eliminate some of them, I can start to home in on the solution. One thing that jumps out are the two squares with the instructions – those must be hidden in the finished product. I try to keep this in mind when I begin folding again.
When I realized I didn’t have to fold along the grid, things got a little crazy.
Theory into practice
The work is not just theoretical – various practical applications of these mathematical folding methods have emerged. Many devices that must start small and expand, like airbags, heart stents, and satellites, have all benefited from origami folding techniques. In fact, NASA has just put out a call for origami designs for a radiation shield.
“Computational origami turned out to be exciting both as a mathematical challenge and in terms of applications to engineering,” says Demaine. He’s currently most excited about folding nanoscale structures to transform 2D nanoprinting technology, like that used in computer chip manufacturing, into technology that can make any 3D shape with nanoscale resolution.
Still, the ability to design any origami structure doesn’t mean it’s easy to turn the flat folding pattern into the final shape. In fact, most complex patterns can’t be constructed one fold at a time and require the simultaneous folding of many creases at once – something called the collapse.
As Demaine tells me, patience is the only requirement to becoming a paper folding expert.
Paper proof
I did eventually solve the 2007 CSAIL puzzle by thinking about the original mathematical problem that it was meant to represent. Indeed, the final solution didn’t emerge fold by fold but came suddenly, as the paper collapsed in on itself from all sides at once. The feeling of satisfaction as I scanned the square and saw the perfect checkerboard is hard to describe. Never have I been so excited to show everyone a folded piece of paper.
I may have solved the puzzle, but the general “turning inside out problem”, which the puzzle represents, remains unsolved. Demaine and other mathematicians working in this field have no shortage of challenges remaining – and plenty of paper possibilities.
–30–


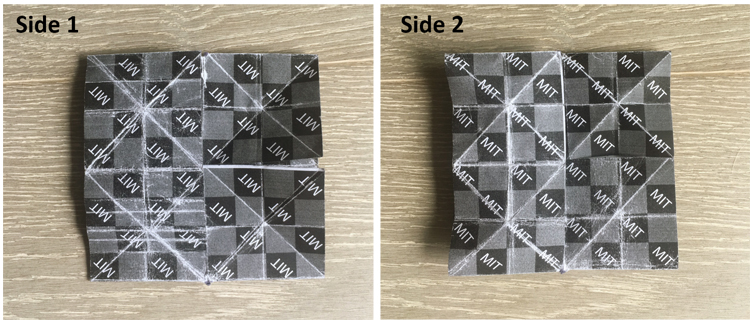



That’s amazing. “The feeling of satisfaction as I scanned the square and saw the perfect checkerboard is hard to describe. Never have I been so excited to show everyone a folded piece of paper.”